[Uh oh, this is sort of disastrously long, practically unedited, and a mixture of tutorial- and expert-level text. Good luck. Send corrections.]

It’s been almost exactly a year since the release of the first Planck cosmology results (which I discussed in some depth at the time). On this auspicious anniversary, we in the cosmology community found ourselves with yet more tantalising results to ponder, this time from a ground-based telescope called BICEP2. While Planck’s results were measurements of the temperature of the cosmic microwave background (CMB), this year’s concerned its polarisation.

Background

Polarisation is essentially a headless arrow that can come attached to the photons coming from any direction on the sky — if you’ve worn polarised sunglasses, and noticed how what you see changes as you rotate them around, you’ve seen polarisation. The same physics responsible for the temperature also generates polarisation. But more importantly for these new results, polarisation is a sensitive probe of some of the processes that are normally mixed in, and so hard to distinguish, in the temperature.

Technical aside (you can ignore the details of this paragraph). Actually, it’s a bit more complicated than that: we can think of the those headless arrows on the sky as the sum of two separate kinds of patterns. We call the first of these the “E-mode”, and it represents patterns consisting of either radial spikes or circles around a point. The other patterns are called the “B-mode” and look like patterns that swirl around, either to the left or the right. The important difference between them is that the E modes don’t change if you reflect them in a mirror, while the B modes do — we say that they have a handedness, or parity, in somewhat more mathematical terms. I’ve discussed the CMB a lot in the past but can’t do the theory of the CMB justice here, but my colleague Wayne Hu has an excellent, if somewhat dated, set of web pages explaining the physics (probably at a physics-major level).

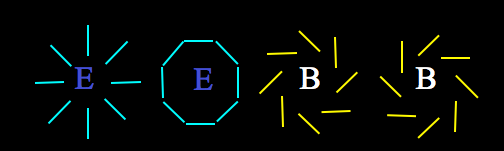
The excitement comes because these B-mode patterns can only arise in a few ways. The most exciting is that they can come from gravitational waves (GWs) in the early Universe. Gravitational waves (sometimes incorrectly called “gravity waves” which historically refers to unrelated phenomena!) are propagating ripples in space-time, predicted in Einstein’s general relativistic theory of gravitation. Because the CMB is generated about 400,000 years after the big bang, it’s only sensitive to gravitational radiation from the early Universe, not astrophysical sources like spiralling neutron stars or — from where we have other, circumstantial, evidence for gravitational waves, and which are the sources for which experiments like LIGO and eLISA will be searching. These early Universe gravitational waves move matter around in a specific way, which in turn induce those specific B-mode polarization pattern.

In the early Universe, there aren’t a lot of ways to generate gravitational waves. The most important one is inflation, an early period of expansion which blows up a subatomically-sized region by something like a billion-billion-billion times in each direction — inflation seems to be the most well thought-out idea for getting a Universe that looks like the one in which we live, flat (in the sense of Einstein’s relativity and the curvature of space-time), more or less uniform, but with small perturbations to the density that have grown to become the galaxies and clusters of galaxies in the Universe today. Those fluctuations arise because the rapid expansion takes minuscule quantum fluctuations and blows them up to finite size. This is essentially the same physics as the famous Hawking radiation from black holes. The fluctuations that eventually create the galaxies are accompanied by a separate set of fluctuations in the gravitational field itself: these are the ones that become gravitational radiation observable in the CMB. We characterise the background of gravitational radiation through the number r, which stands for the ratio of these two kinds of fluctuations — gravitational radiation divided by the density fluctuations.

Important caveat: there are other ways of producing gravitational radiation in the early Universe, although they don’t necessarily make exactly the same predictions; some of these issues have been discussed by my colleagues in various technical papers (Brandenberger 2011; Hindmarsh et al 2008; Lizarraga et al 2014 — the latter paper from just today!).

However, there are other ways to generate B modes. First, lots of astrophysical objects emit polarised light, and they generally don’t preferentially create E or B patterns. In particular, clouds of gas and dust in our galaxy will generally give us polarised light, and as we’re sitting inside our galaxy, it’s hard to avoid these. Luckily, we’re towards the outskirts of the Milky Way, so there are some clean areas of sky, but it’s hard to be sure that we’re not seeing some such light — and there are very few previous experiments to compare with.

We also know that large masses along the line of sight — clusters of galaxies and even bigger — distort the path of the light and can move those polarisation arrows around. This, in turn, can convert what started out as E into B and vice versa. But we know a lot about that intervening matter, and about the E-mode pattern that we started with, so we have a pretty good handle on this. There are some angular scales over which this is larger than the gravitational wave signal, and some scales that the gravitational wave signal is dominant.

So, if we can observe B-modes, and we are convinced that they are primordial, and that they are not due to lensing or astrophysical sources, and they have the properties expected from inflation, then (and only then!) we have direct evidence for inflation!

Data

Here’s a plot, courtesy the BICEP2 team, with the current state of the data targeting these B modes:

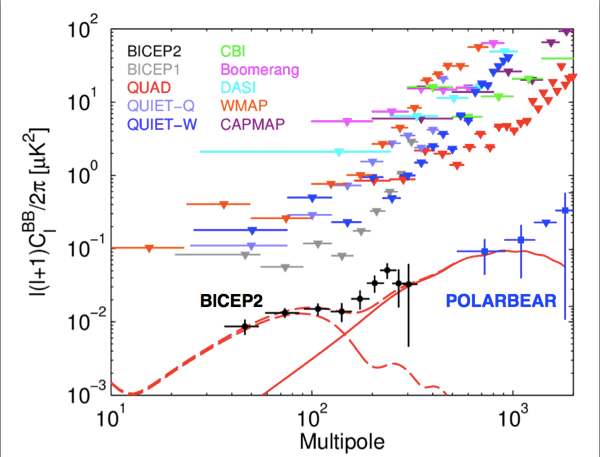
The figure shows the so-called power spectrum of the B-mode data — the horizontal “multipole” axis corresponds to angular sizes (θ) on the sky: very roughly, multipole ℓ ~ 180°/θ. The vertical axis gives the amount of “power” at those scales: it is larger if there are more structures of that particular size.
The downward pointing arrows are all upper limits; the error bars labeled BICEP2 and Polarbear are actual detections. The solid red curve is the expected signal from the lensing effect discussed above; the long-dashed red curve is the effect of gravitational radiation (with a particular amplitude), and the short-dashed red curve is the total B-mode signal from the two effects.

The Polarbear results were announced on 11 March (disclosure: I am a member of the Polarbear team). These give a detection of the gravitational lensing signal. It was expected, and has been observed in other ways both in temperature and polarisation, but this was the first time it’s been seen directly in this sort of B-mode power spectrum, a crucial advance in the field, letting us really see lensing unblurred by the presence of other effects. We looked at very “clean” areas of the sky, in an effort to minimise the possible contamination from those astrophjysical foregrounds.

The BICEP2 results were announced with a big press conference on 17 March. There are two papers so far, one giving the scientific results, another discussing the experimental techniques used — more papers discussing the data processing and other aspects of the analysis are forthcoming. But there is no doubt from the results that they have presented so far that this is an amazing, careful, and beautiful experiment.

Taken at face value, the BICEP2 results give a pretty strong detection of gravitational radiation from the early Universe, with the ratio parameter r=0.20, with error bars +0.07 and -0.05 (they are different in the two different directions, so you can’t write it with the usual “±”).

This is why there has been such an amazing amount of interest in both the press and the scientific community about these results — if true, they are a first semi-direct detection of gravitational radiation, strong evidence that inflation happened in the early Universe, and therefore a first look at waves which were created in the first tiny fraction of a second after the big bang, and have been propagating unimpeded in the Universe ever since. If we can measure more of the properties of these waves, we can learn more about the way inflation happened, which may in turn give us a handle on the particle physics of the early Universe and ultimately on a so-called “theory of everything” joining up quantum mechanics and gravity.

Taken at face value, the BICEP2 results imply that the very simplest theories of inflation may be right: the so-called “single-field slow-roll” theories that postulate a very simple addition to the particle physics of the Universe. In the other direction, scientists working on string theory have begun to make predictions about the character of inflation in their models, and many of these models are strongly constrained — perhaps even ruled out — by these data.

Skepticism

This is great. But scientists are skeptical by nature, and many of us have spent the last few days happily trying to poke holes in these results. My colleagues Peter Coles and Ted Bunn have blogged their own worries over the last couple of days, and Antony Lewis has already done some heroic work looking at the data.

The first worry is raised by their headline result: r=0.20. On its face, this conflicts with last year’s Planck result, which says that r<0.11 (of course, both of these numbers really represent probability distributions, so there is no absolute contradiction between these numbers, but rather they should be seen to be as a very unlikely combination). How can we ameliorate the “tension” (a word that has come into vogue in cosmology lately: a wimpy way — that I’ve used, too — of talking about apparent contradictions!) between these numbers?

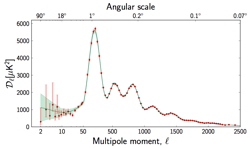First, how does Planck measure r to begin with? Above, I wrote about how B modes show only gravitational radiation (and lensing, and astrophysical foregrounds). But the same gravitational radiation also contributes to the CMB temperature, albeit at a comparatively low level, and at large angular scales — the very left-most points of the temperature equivalent of a plot like the above — I reproduce one from last year’s Planck release at right. In fact, those left-most data points are a bit low compared to the most favoured theory (the smooth curve), which pushes the Planck limit down a bit.

But Planck and BICEP2 measure r at somewhat different angular scales, and so we can “ameliorate the tension” by making the theory a bit more complicated: the gravitational radiation isn’t described by just one number, but by a curve. If both data are to be believed, the curve slopes up from the Planck regime toward the BICEP2 regime. In fact, such a new parameter is already present in the theory, and goes by the name “tensor tilt”. The problem is that the required amount of tilt is somewhat larger than the simplest ideas — such as the single-field slow-roll theories — prefer.

If we want to keep the theories simple, we need to make the data more complicated: bluntly, we need to find mistakes in either Planck or BICEP2. The large-scale CMB temperature sky has been scrutinised for the last 20 years or so, from COBE through WMAP and now Planck. Throughout this time, the community has been building up a catalog of “anomalies” (another term of art we use to describe things we’re uncomfortable with), many of which do seem to affect those large scales. The problem is that no one quite figure out if these things are statistically significant: we look at so many possible ways that the sky could be weird, but we only publish the ones that look significant. As my Imperial colleague Professor David Hand would point out, “Coincidences, Miracles, and Rare Events Happen Every Day“. Nonetheless, there seems to be some evidence that something interesting/unusual/anomalous is happening at large scales, and perhaps if we understood this correctly, the Planck limits on r would go up.

But perhaps not: those results have been solid for a long while without an alternative explanation. So maybe the problem is with BICEP2? There are certainly lots of ways they could have made mistakes. Perhaps most importantly, it is very difficult for them to distinguish between primordial perturbations and astrophysical foregrounds, as their main results use only data from a single frequency (like a single colour in the spectrum, but down closer to radio wavelengths). They do compare with some older data at a different frequency, but the comparison does not strongly rule out contamination. They also rely on models for possible contamination, which give a very small contribution, but these models are very poorly constrained by current data.

Another way they could go wrong is that they may misattribute some of their temperature measurement, or their E mode polarisation, to their B mode detection. Because the temperature and E mode are so much larger than the B they are seeing, only a very small amount of such contamination could change their results by a large amount. They do their best to control this “leakage”, and argue that its residual effect is tiny, but it’s very hard to get absolutely right.

And there is some internal evidence within the BICEP2 results that things are not perfect. The most obvious one comes from the figure above: the points around &ell;=200 — where the lensing contributions begins to dominate — are a bit higher than the model. Is this just a statistical fluctuation, or is it evidence of a broader problem? Their paper show some somewhat discrepant points in their E polarisation measurements, as well. None of these are very statistically significant, and some may be confirmed by other measurements, but there are enough of these that caution makes sense. From only a few days thinking about the results (and not yet really sitting down and going through the papers in great depth), it’s hard to make detailed judgements. It seems like the team have been careful that it’s hard to imagine the results going away completely, but easy to imagine lots of ways in which it could be wrong in detail.

But this skepticism from me and others is a good thing, even for the BICEP2 team: they will want their results scrutinised by the community. And the rest of us in the community will want the opportunity to reproduce the results. First, we’ll try to dig into the BICEP2 results themselves, making sure that they’ve done everything as well as possible. But over the next months and years, we’ll want to reproduce them with other experiments.

First, of course, will be Planck. Since I’m on Planck, there’s not much I can say here, except that we expect to release our own polarisation data and cosmological results later this year. This paper (Efstathiou and Gratton 2009) may be of interest….

Next, there are a bunch of ground- and balloon-based CMB experiments gathering data and/or looking for funding right now. The aforementioned Polarbear will continue, and I’m also involved with the EBEX team which hopes to fly a new balloon to probe the CMB polarisation again in a few years. In the meantime, there’s also ACT, SPIDER, SPT, and indeed the successor to BICEP itself, called the Keck array, and many others besides. Eventually, we may even get a new CMB satellite, but don’t hold your breath…

Rumour-mongering

I first heard about the coming BICEP2 results in the middle of last week, when I was up in Edinburgh and received an email from a colleague just saying “r=0.2?!!?” I quickly called to ask what he meant, and he transmitted the rumour of a coming BICEP detection, perhaps bolstered by some confirmation from their successor experiment, the Keck Array (which does in fact appear in their paper). Indeed, such a rumour had been floating around the community for a year or so, but most of thought it would turn out to be spurious. But very quickly last week, we realised that this was for real. It became most solid when I had a call from a Guardian journalist, who managed to elicit some inane comments from me, before anything was known for sure.

By the weekend, it became clear that there would be an astronomy-related press conference at Harvard on Monday, and we were all pretty sure that it would be the BICEP2 news. The number r=0.20 was most commonly cited, and we all figured it would have an error bar around 0.06 or so — small enough to be a real detection, but large enough to leave room for error (but I also heard rumours of r=0.075).

By Monday morning, things had reached whatever passes for a fever pitch in the cosmology community: twitter and Facebook conversations, a mention on BBC Radio 4’s Today programme, all before the official title of the press conference was even announced: “First Direct Evidence for Cosmic Inflation“. Apparently, other BBC journalists had already had embargoed confirmation of some of the details from the BICEP2 team, but the embargo meant they couldn’t participate in the rumour-spreading.

I was traveling during most of this time, fielding occasional call from journalists (there aren’t that many CMB-specialists within within easy of the London-based media), though, unfortunately for my ego, I wasn’t able to make it onto any of Monday night’s choice tv spots.

By the time of the press conference itself, the cosmology community had self-organised: there was a Facebook group organised by Fermilab’s Scott Dodelson, which pretty quickly started dissecting the papers and was able to follow along with the press conference as it happened (despite the fact that most of us couldn’t get onto the website — one of the first times that the popularity of cosmology has brought down a server).

At the time, I was on a series of trains from Loch Lomond to Glasgow, Edinburgh and finally on to London, but the facebook group made (from a tech standpoint, it’s surprising that we didn’t do this on the supposedly more capable Google Plus platform, but the sociological fact is that more of us are on, and use, Facebook). It was great to be able to watch, and participate in, the real-time discussion of the papers (which continues on Facebook as of now). Cosmologists have been teasing out possible inconsistencies (some of which I alluded to above), trying to understand the implications of the results if they’re right — and thinking about the next steps. IRL, Now that I’m back at Imperial, we’ve been poring over the papers in yet more detail, trying to work exactly how they’ve gathered and analysed their data, and seeing what parts we want to try to reproduce.

Aftermath

Physics moves fast nowadays: as of this writing, about 72 hours after the announcement, there are 16 papers mentioning the BICEP2 results on the physics ArXiV (it’s a live search, so the number will undoubtedly grow). Most of them attempt to constrain various early-Universe models in the light of the r=0.20 results — some of them with some amount of statistical rigour, others just pointing out various models in which that is more or less easy to get. (I’ve obviously spent too much time on this post and not enough writing papers.)

It’s also worth collecting, if only for my own future reference, some of the media coverage of the results:

• The BBC’s excellent news piece and nice explanatory supplement
• The Wall Street Journal
• The Guardian
• The Telegraph
• The Economist
• IEEE Spectrum (on the more technical side)

For more background, you can check out

• Sean Carroll’s introduction and post-press-conference debrief
• Peter Coles’ liveblog, straw poll, and skeptical summary